How many sig figs in 0.0001 – Embarking on an intriguing exploration, we delve into the depths of significant figures, seeking to unravel the mystery of how many reside within the enigmatic 0.0001. Join us as we navigate the intricacies of this concept, uncovering its significance in scientific calculations and beyond.
In this comprehensive guide, we’ll illuminate the concept of significant figures, demonstrating how to count them in 0.0001. We’ll explore the role of leading and trailing zeros, examining why the zeros in 0.0001 hold significance. Delving further, we’ll investigate the relationship between scientific notation and significant figures, exploring the implications for accurate calculations.
Significant Figures in 0.0001
In the realm of science and measurement, significant figures, often abbreviated as sig figs, hold immense importance. They represent the digits in a number that are known with certainty and convey the precision of a measurement.
To determine the sig figs in 0.0001, let’s delve into the concept further.
Counting Significant Figures
The counting process hinges on the following rules:
- All non-zero digits are significant.
- Zeros between non-zero digits are significant.
- Trailing zeros to the right of a decimal point, with no decimal point to the left, are not significant.
- Leading zeros to the left of the first non-zero digit are not significant.
Applying these rules to 0.0001, we find:
- The first non-zero digit is 1, which is significant.
- The zeros between the 1 and the decimal point are significant.
- The trailing zeros to the right of the decimal point are not significant.
Therefore, 0.0001 has twosignificant figures.
Leading and Trailing Zeros

Leading and trailing zeros play a crucial role in determining significant figures. Leading zeros, which appear before the first non-zero digit, are never significant. On the other hand, trailing zeros, which follow the last non-zero digit, are significant if there is a decimal point present.
In the case of 0.0001, the zeros are significant because there is a decimal point present. This means that 0.0001 has two significant figures, which are the digits 1 and the zero immediately to its left.
Distinction between Significant and Non-Significant Zeros, How many sig figs in 0.0001
To further clarify the distinction between significant and non-significant zeros, consider the following examples:
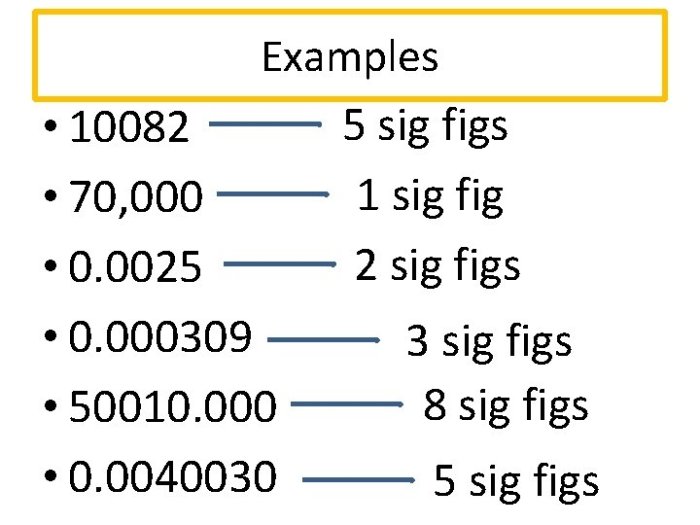
- 0.005 has two significant figures (5 and 0).
- 0.0005 has three significant figures (5, 0, and 0).
- 500 has two significant figures (5 and 0), even though there are trailing zeros.
- 0.500 has three significant figures (5, 0, and 0) because there is a decimal point present.
Scientific Notation and Sig Figs

Scientific notation is a way of expressing very large or very small numbers in a more compact and manageable form. It is especially useful in scientific calculations, where numbers with many significant figures are often encountered.
The general form of scientific notation is:
a × 10n
If you’re pondering how many significant figures are lurking within the minuscule 0.0001, fear not! This numerical enigma can be solved with a simple trick. Just like the detailed steps outlined in this oral mech exam write up , understanding the concept of significant figures is a piece of cake.
Dive in and unravel the secrets of 0.0001’s hidden precision!
where:
- a is a number between 1 and 10 (inclusive)
- n is an integer
For example, the number 0.0001 can be written in scientific notation as:
1 × 10-4
This means that the decimal point has been moved four places to the right, and the exponent is -4. The number 1 is the coefficient, and it is always written without a decimal point.
When converting a number to scientific notation, it is important to make sure that the number of significant figures is preserved. In the case of 0.0001, there is only one significant figure, so the scientific notation must also have only one significant figure.
This means that the coefficient must be rounded to 1.
Scientific notation can be a useful tool for managing significant figures in calculations. By keeping track of the number of significant figures in the coefficient and the exponent, it is possible to ensure that the final answer has the correct number of significant figures.
Applications of Sig Figs in Calculations

Significant figures play a crucial role in calculations as they help maintain the accuracy of the final result. By considering the number of significant figures in the initial values, we can ensure that the calculated result is meaningful and reliable.
When adding or subtracting numbers with different significant figures, the result should be rounded to the least number of decimal places among the numbers being combined. For instance, if we add 2.30 (three significant figures) and 1.2 (two significant figures), the result should be reported as 3.5 (two significant figures).
In multiplication and division, the result should be reported with the same number of significant figures as the number with the fewest significant figures. For example, if we multiply 2.30 (three significant figures) by 1.200 (four significant figures), the result should be 2.76 (three significant figures).
Expert Answers: How Many Sig Figs In 0.0001
What are significant figures?
Significant figures are digits in a number that are known with certainty and convey meaningful information about the quantity being measured.
How many significant figures are in 0.0001?
There are 2 significant figures in 0.0001: the 1 and the first 0.
Why are the zeros in 0.0001 significant?
The zeros in 0.0001 are significant because they indicate the place value of the 1. Without these zeros, the number would be 0.1, which has only one significant figure.