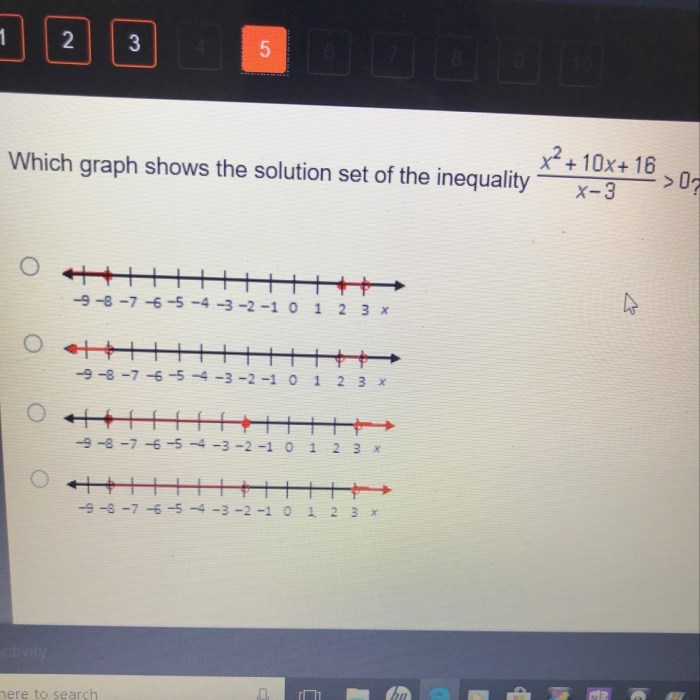
Graph the solution set to this inequality. 3x-11 7x+9 – Delving into the concept of graphing the solution set to the inequality 3x-11< 7x+9, we embark on a mathematical journey that unveils the intricacies of this algebraic expression. Through a systematic approach, we will explore the boundaries that define the solution set, unravel the points of intersection, and ultimately determine the region that satisfies the inequality.
By delving into the nuances of this inequality, we not only gain a deeper understanding of its graphical representation but also hone our problem-solving skills, fostering a critical and analytical mindset.
Graphing the Solution Set of an Inequality
Inequalities are mathematical expressions that represent a relationship between two values or expressions, where one value is less than, greater than, or equal to the other. To graph the solution set of an inequality, we follow a step-by-step process that involves creating a coordinate plane, plotting the boundary lines of the inequality, shading the appropriate region, and testing points within the regions.
1. Graph the Solution Set
To graph the solution set of an inequality, we first create a coordinate plane with x- and y-axes. Next, we plot the boundary lines of the inequality, which are the lines that represent the equations 3x – 11 = 0 and 7x + 9 = 0. The region that satisfies the inequality is the region that lies on one side of the boundary lines.
In this case, the region that satisfies the inequality 3x – 11< 7x + 9 is the region that lies below the line 3x - 11 = 0.
2. Determine the Points of Intersection, Graph the solution set to this inequality. 3x-11 7x+9
To determine the points of intersection between the boundary lines, we solve the system of equations 3x – 11 = 0 and 7x + 9 = 0. Solving this system gives us the points (-1, -2) and (-3/7, -12/7). These points divide the coordinate plane into three regions: the region above the line 3x – 11 = 0, the region below the line 7x + 9 = 0, and the region between the two lines.
3. Test Points in the Regions
To determine which region satisfies the inequality, we test points in each region. For example, we can test the point (0, 0) in the region above the line 3x – 11 = 0. Substituting the coordinates of the point into the inequality gives us 3(0) – 11< 7(0) + 9, which simplifies to -11 < 9. This inequality is true, so the point (0, 0) lies in the solution set of the inequality.
4. Conclusion
The solution set of the inequality 3x – 11< 7x + 9 is the region that lies below the line 3x - 11 = 0. This region is the shaded region in the coordinate plane that lies below the line y = (3/7)x - 12/7.
Question & Answer Hub: Graph The Solution Set To This Inequality. 3x-11 7x+9
What is the significance of graphing the solution set to an inequality?
Graphing the solution set provides a visual representation of the inequality, making it easier to identify the region that satisfies the inequality and understand the relationship between the variables.
How do I determine the points of intersection for the boundary lines?
To find the points of intersection, solve the system of equations formed by setting each boundary line equation equal to zero.
What is the role of test points in determining the solution set?
Test points are used to verify whether a given region satisfies the inequality by substituting the coordinates of the point into the inequality and checking if it holds true.